In 1973, as a part of a series on Three Way Interacting Great Circles (the foundational geometry for the geodesic dome), Buckminster Fuller made this simple but prophetic note:
Omnitriangulated geodesic spheres consisting exclusively of three-way interacting great circles are realizations of gravitational field patterns. The gravitational field will ultimately be disclosed as ultra high-frequency tensegrity geodesic spheres. Nothing else.
R. Buckminster Fuller
As we have come to understand the geometry of gravity in recent Unified Physics breakthroughs, including “Quantum Gravity and the Holographic Mass” by Nassim Haramein, we see that Buckminster Fuller may have been exactly accurate. At the quantum scale, gravity can be seen to arise from geometric permutations of the quantum field. The “architecture” of the quantum field must be in extremely high equilibrium, suggesting a tetrahedral packing mechanism, which Buckminster Fuller often described as the “building blocks of the Universe.”
In Nassim Haramein’s theory, each node in the geodesic (or point on the tetrahedron grid) is a Planck Spherical Unit. These spheres are interlocking and intersecting, space-filling. However, intersecting spheres follow the same rules as tetrahedral grids (or hexagonal grids), and so the same fundamental geometry of spacetime arises in either case.
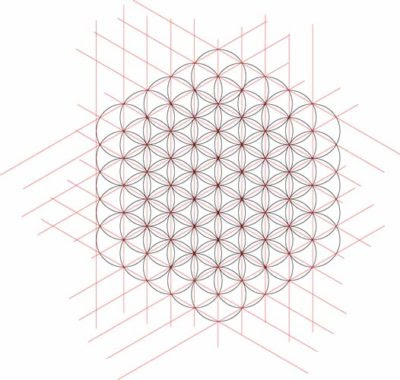
Flower of Life and Triangulation Patterns
If we take a slice of this spherical or tetrahedral grid, we see the ancient pattern of the flower of life, and a simple arrangement of “three-way” triangular grids.
The Geometry of Quantum Gravity, show that this geometric form is in total equilibrium. This means that there is ZERO locally generated gravity when spacetime is in this arrangement… By locally generated, we mean that the spacetime fabric in this position is not creating any additional tension or force of gravity, which arises due to the curvature of spacetime, as Einstein described it. However, nearly every point in spacetime is part of a larger gravity well. Consider that we are currently in the gravity well of the Earth, Moon, Sun, Galaxy, Laniakea Cluster, and whatever larger gravitational structures exist in the Universe.
All of these gravity wells occur due to spacetime curvature around a central node. These central nodes are the only points that cannot in themselves be said to have curvature, and so we might quite accurately define them as “singularities.” They are points at which curvature becomes infinite, like the point in the center of any regular solid or sphere.
Returning to Buckminster Fuller’s quote, let’s break it down in context:
“Omnitriangulated geodesic spheres…”
This refers to the equilateral triangle structured placement of all nodes in the quantum field (for maximum efficiency and equilibrium).
“…consisting exclusively of three-way interacting great circles…”
This means that there are continuous lines of energy running through any plane or surface of the geometric structure, such as the event horizon of a black hole or charge radius of a proton. These energy lines intersect in triangulation (like a tetrahedron), interacting with tension in those positions.
“…are realizations of gravitational field patterns.”
This is a description of how gravitational fields are actually patterned.
“The gravitational field will ultimately be disclosed as ultra high-frequency tensegrity geodesic spheres. Nothing else.”
Ultra high-frequency here refers to the Planck scale, which is the highest known frequency scale of the electromagnetic field. The tensegrity is the tension formed between the Planck Spherical Units (PSU) or quantum nodes attempting to return to total equilibrium. Because of this tensegrity, the geometries formed by these PSU exactly match the tension patterns in geodesic spheres.
Buckminster Fuller was right!
Adam Apollo


Sem comentários:
Enviar um comentário